Scientists use the Periodic Table in order to find out important
information about various elements. Created by Dmitri Mendeleev
(1834-1907), the periodic table orders all known elements in accordance
to their similarities. When Mendeleev began grouping elements, he
noticed the Law of Chemical Periodicity. This law states, "the
properties of the elements are periodic functions of atomic number."
The periodic table is a chart that categorizes elements by "groups" and
"periods."
All elements are ordered by their atomic number. The atomic
number is the number of protons per atom. In a neutral atom, the number
of electrons equals the number of protons. The periodic table
represents neutral atoms.
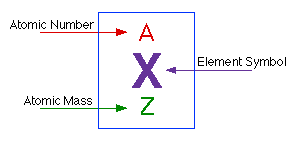
The atomic number is typically located above the element symbol.
Beneath the element symbol is the atomic mass. Atomic mass is
measured in Atomic Mass Units where 1 amu = (1/12) mass of carbon
measured in grams. The atomic mass number is equal to the number of
protons plus neutrons, which provides the average weight of all isotopes
of any given element. This number is typically found beneath the
element symbol. Atoms with the same atomic number, but different mass
numbers are called isotopes. Below is a diagram of a typical cells on
the periodic table.
There are two main classifications in the periodic table, "groups" and "periods." Groups are the vertical columns that include elements with similar chemical and physical properties. Periods are the horizontal rows. Going from left to right on the periodic table, you will find metals, then metalloids, and finally nonmetals. The 4th, 5th, and 6th periods are called the transition metals. These elements are all metals and can be found pure in nature. They are known for their beauty and durability. The transition metals include two periods known as the lanthanides and the actinides, which are located at the very bottom of the periodic table. The chart below gives a brief description of each group in the periodic table.
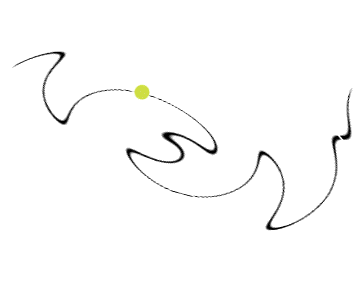
Chemists care about where electrons are in an atom or a molecule. In the early models, we believed that electrons move like billiard balls, and followed the rules of classical physics. The graphic below attempts to show that earlier models thought that we could identify the exact path, position, velocity, etc. of an electron or electrons in an atom:
A more accurate picture is that the electron(s) reside in a "cloud" that
surrounds the nucleus of the atom. This concept is shown in the
graphic below:

Chemists are interested in predicting the probability that the electron will be at some particular part of this cloud. The cloud is better known as an orbital,
and comes in several different types, or shapes. Atomic orbitals are
known as s, p, d, and f orbitals. Each type of atomic orbital has
certain characteristics, such as shape. For example, as the graphic
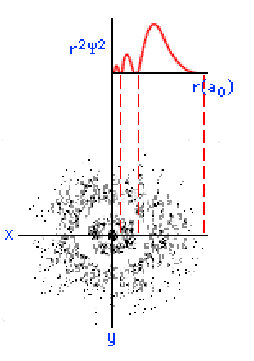
below shows, an s orbital is spherical in shape:
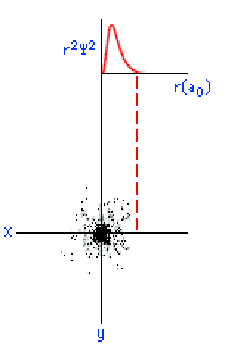
On this graph, the horizontal (x) axis represents the distance from the nucleus in units of a0, or atomic units. The value of a0
is 0.0529 nanometers (nm). The vertical (y) axis represents the
probability density. What you should
notice is that as the electron moves farther away from the nucleus, the
probability of its being found at that distance decreases. In other
words, the electron prefers to hang around close to the nucleus.
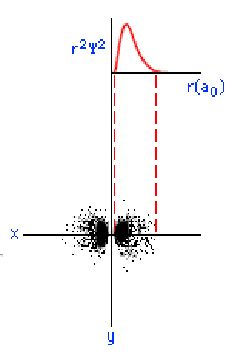
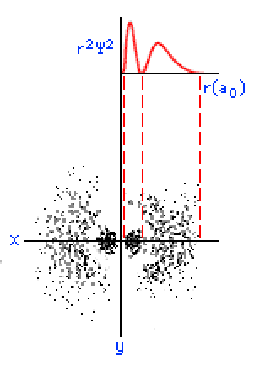
The three graphics below show some other orbitals. The first graph (top left) is of a "2s" orbital. Each "s" orbital can hold two electrons in its cloud. Notice how there is a relatively high probability of an electron being near the nucleus, then some space where the probability is close to zero, then the probability increases substantially at some distance from the nucleus. The graphic at the top right shows a "2p" atomic orbital. Orbitals that are "p" orbitals can hold up to six (6) electrons in their cloud. Notice its "dumbbell" or "figure of eight" shape. At the bottom left is a "3s" orbital. Again, notice its spherical shape. Finally, at the bottom right, is a "3p" orbital.

The table below shows the subshells, the number of orbitals, and the maximum number of electrons allowed:
The Abridged (shortened) Periodic Table below shows the electron
configurations of the elements. Notice for space reasons we sometimes
leave off a portion of the electron configuration. For example, look at
argon (Ar), element 18. The table below shows
its electron configuration as "[3s23p6]" (remembering that "p" orbitals can hold up to six (6) electrons). Its actual electron configuration is:
Ar = [1s22s22p63s23p6]
Sometimes you will see the notation: "[Ne]3s23p6", which means to include everything that is in neon (Ne, 10) plus the stuff in the "3"-level orbitals

There are two main classifications in the periodic table, "groups" and "periods." Groups are the vertical columns that include elements with similar chemical and physical properties. Periods are the horizontal rows. Going from left to right on the periodic table, you will find metals, then metalloids, and finally nonmetals. The 4th, 5th, and 6th periods are called the transition metals. These elements are all metals and can be found pure in nature. They are known for their beauty and durability. The transition metals include two periods known as the lanthanides and the actinides, which are located at the very bottom of the periodic table. The chart below gives a brief description of each group in the periodic table.
| Group 1A |
|
| Group 2A |
|
| Group 3A |
|
| Group 4A |
|
| Group 5A |
|
| Group 6A |
|
| Group 7A |
|
| Group 8A |
|
Charges in the Atom
The charges in the atom are crucial in understanding how the atom works. An electron has a negative charge, a proton has a positive charge and a neutron has no charge. Electrons and protons have the same magnitude of charge. Like charges repel, so protons repel one another as do electrons. Opposite charges attract which causes the electrons to be attracted to the protons. As the electrons and protons grow farther apart, the forces they exert on each other decrease.Atomic Models and the Quantum Numbers
There are different models of the structure of the atom. One of the first models was created by Niels Bohr, a Danish physicist. He proposed a model in which electrons circle the nucleus in "orbits" around the nucleus, much in the same way as planets orbit the sun. Each orbit represents an energy level which can be determined using equations generated by Planck and others discussed in more detail below. The Bohr model was later proven to be incorrect, but provides a useful model for building an explanation. The "accepted" model is the quantum model. In the quantum model, we state that the electron cannot be found precisely, but we can predict the probability, or likelihood, of an electron being at some location in the atom. You should be familiar with quantum numbers, a series of three numbers used to describe the location of some object (like an electron) in three-dimensional space:- n: the principal quantum number, an integer value (1, 2, 3...) that
is used to describe the quantum level, or shell, in which an electron
resides. The principal quantum number is the primary number used to
determine the amount of energy in an atom. Using one of the first
important equations in atomic structure (developed by Niels Bohr), we
can calculate the amount of energy in an atom with an electron at some
value of n:
En = - Rhc
n2
R = Rydberg constant, a value of 1.097 X 107 m-1
c = speed of light, 3.00 X 108 m/s
h = Planck's constant, 6.63 X 10 -34 J-s
n = principal quantum number, no unit For example, how much energy does one electron with a principal quantum number of n= 2 have?
En = - Rhc
n2or En = - (1.097x107 m-1 ∗ (6.63x10-34 J•s)∗(3.0x108 m•s-1)
22= 5.5x10-19 J
ΔE = hv = RH 1
ni2- 1
nf2
ΔE = change in energy (Joules)
h = Planck's constant with a value of 6.63 x 10-34 (J-s)
ν is frequency (s-1)
RH is the Rydberg constant with a value of 2.18 x 10-18J.
ni is the initial quantum number
nf is the final quantum number
Using the equation below, we can calculate the wavelength and the frequency of the energy. The wavelength and the frequency give us information about how we might "see" the energy:
vλ = c
ν = the frequency of radiation (s-1)
λ = the wavelength (m)
c = the speed of light with a value of 3.00 x 108 m/s in a vacuum
Speed of light = 3.00E+08 Rydberg constant = 2.18E-18 Planck's constant = 6.63E-34 Excited state, n = 3 4 5 Ground state, n = 2 2 2 Excited state energy (J) 2.42222E-19 1.363E-19 8.72E-20 Ground state energy (J) 5.45E-19 5.45E-19 5.45E-19 ΔE = -3.02778E-19 -4.09E-19 -4.58E-19 ν = 4.56678E+14 6.165E+14 6.905E+14 λ(nm) = 656.92 486.61 434.47 - l ("el", not the number 1): the azimuthal quantum
number, a number that specifies a sublevel, or subshell, in an orbital.
The value of the azimuthal quantum number is always one less than the
principal quantum number n. For example, if n=1, then "el"=0. If n=3,
then l can have three values: 0,1, and 2. The values of l
are typically not identified as "0, 1, 2, and 3" but are more commonly
called by their historic names, "s, p, d, and f", respectively. Since
the quantum numbers were discovered through the study of light and lines
on an electromagnetic spectra, chemists identified the lines by their
quality: sharp, principal, diffuse and fundamental. The table below shows the relationship:
Value of l Subshell designation 0 s 1 p 2 d 3 f - m: the magnetic quantum number. Each subshell is composed
of one or more orbitals. In the study of light, it was discovered that
additional lines appeared in the spectra produced when light was
emitted in a magnetic field. The magnetic quantum number has values
between -l and +l. When l =1, for example, m can
have three values: -1, 0, and +1. Because you know from the chart
above that the subshell designation for l =1 is "p", you now know that the p orbital has three components. In your study of chemistry, you will be presented with px, py, and pz.
Notice how the subscripts are related to a three-dimensional
coordinate system, x, y, and z. The chart below shows a summary of
the quantum numbers:
Principal Quantum Number (n) Azimuthal Quantum Number (l) Subshell Designation Magnetic Quantum Number (m) Number of orbitals in subshell 1 0 1s 0 1 2 0
12s
2p0
-1 0 +11
33 0
1
23s
3p
3d0
-1 0 +1
-2 -1 0 +1 +21
3
54 0
1
2
34s
4p
4d
4f0
-1 0 +1
-2 -1 0 +1 +2
-3 -2 -1 0 +1 +2 +31
3
5
7
Chemists care about where electrons are in an atom or a molecule. In the early models, we believed that electrons move like billiard balls, and followed the rules of classical physics. The graphic below attempts to show that earlier models thought that we could identify the exact path, position, velocity, etc. of an electron or electrons in an atom:



The three graphics below show some other orbitals. The first graph (top left) is of a "2s" orbital. Each "s" orbital can hold two electrons in its cloud. Notice how there is a relatively high probability of an electron being near the nucleus, then some space where the probability is close to zero, then the probability increases substantially at some distance from the nucleus. The graphic at the top right shows a "2p" atomic orbital. Orbitals that are "p" orbitals can hold up to six (6) electrons in their cloud. Notice its "dumbbell" or "figure of eight" shape. At the bottom left is a "3s" orbital. Again, notice its spherical shape. Finally, at the bottom right, is a "3p" orbital.




Determining Electron Configuration
One of the skills you will need to learn to succeed in freshman chemistry is being able to determine the electron configuration of an atom. An electron configuration is basically an account of how many electrons there are, and in what orbitals they reside under "normal" conditions. For example, the element hydrogen (H) has one electron. We know this because its atomic number is one (1), and the atomic number tells you the number of electrons. Where does this electron go? The one electron of hydrogen goes into the lowest energy state it possibly can, which means it will start at "level" one and goes into "s" orbitals first. We say that hydrogen has a "[1s1]" electron configuration. Looking at the next element on the Periodic Table --helium, or He -- we see it has an atomic number of two, so two electrons. Since " s" orbitals can hold up to two electrons, helium has an electron configuration of "[1s2]". What about larger atoms? Let's look at carbon, with an atomic number of 6. Where do its 6 electrons go?- First two: 1s2
- Next two: 2s2
- Last two: 2p2
The table below shows the subshells, the number of orbitals, and the maximum number of electrons allowed:
| Subshell | Number of Orbitals | Maximum Number of Electrons |
|---|---|---|
| s | 1 | 2 |
| p | 3 | 6 |
| d | 5 | 10 |
| f | 7 | 14 |
No comments:
Post a Comment