Before a protein or other biological macromolecule can be
rigorously studied from a structural and functional basis, it must be purified. The
problems that can arise during protein purification become clear when one considers that a
single protein has to be purified from a mixture of as many 10,000 proteins, each of which
are made up of the same constituent amino acids. Proteins differ in size (how many amino
acids), charge (how many positively and negatively charged amino acids), and in sequence
and presence of specific binding sites on the proteins. Any technique that could be used
to purify protein must be based on these inherent differences. Three major column chromatography techniques used to separate
proteins (or other macromolecules) will be discussed below.
Proteins of different sizes are separated on a column in which the stationary phase is an polymerized agarose or acrylamide bead, which contain pores of various sizes. A smaller protein in the mobile phase (aqueous buffered solution) may enter the pores in the bead, while a larger protein may not, due to size restriction. The result is that a larger fraction of the overall volume of the column is available to the smaller protein, which thus spends a longer time on the column and is eluted by the mobile solvent after the larger protein. The shape of the protein (not only its size) also determines whether the protein will enter the pores. We used this technique to separate dye encapsulated in liposomes from free dye in Lab 1.
Lets consider in more depth the theory of gel filtration chromatography. Several different column volumes can be defined as shown below.
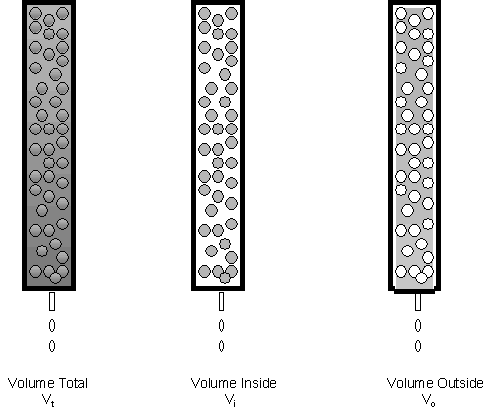 If we consider the mass of the beads to offer a
negligible amount to the volume of the bead, the actual volume in the bead
represents trapped solution and the "stationary" phase. The
volume around the bead is called the void volume, Vo.
Also, Vi = Vt - Vo.
If we consider the mass of the beads to offer a
negligible amount to the volume of the bead, the actual volume in the bead
represents trapped solution and the "stationary" phase. The
volume around the bead is called the void volume, Vo.
Also, Vi = Vt - Vo.
A solute elutes from the column in a broad peak. If the sample volume applied to the column is very small compared to Vt, the volume at which a solute elutes, Ve, is considered to be the center of the elution peak. This is true when Vsample << Ve.
If we view this chromatography has a partitioning of solute between the mobile and stationary phases, we might be interested in what fraction of the stationary phase, Vi, a solute might partition into. Such a ratio would be given by:
The chromatography resin in this type of chromatography consist of an agarose, acrylamide, or cellulose resin or bead which is derivatized to contain covalently linked positively or negatively charged groups. Proteins in the mobile phase will bind through electrostatic interactions to the charged group on the column. In a mixture of proteins, positively charged proteins will bind to a resin containing negatively charged groups, like the carboxymethyl group, CM (-OCH2COO-) or sulfopropyl, SP, (-OCH2CH2CH2SO3-) while the negatively charged proteins will pass through the column. The positively charged proteins can be eluted from the column with a mobile phase containing either a gradient of increasing salt concentration or a single higher salt concentration (isocratic elution). The most positively charged protein will be eluted last, at the highest salt concentration. Likewise, negatively charged proteins will bind to a resin containing positively charged groups, like the diethylaminoethyl group, DEAE (-OCH2CH2NH(C2H5)2+) or a quarternary ethyl amino group, QAE, and can be separated in an analogous fashion. In chromatofocusing, the mobile phase contains a gradient in pH, and bound proteins are eluted when the pH reaches the isoelectric point of the protein, at which the proteins have no net charge. In this lab, you will use QAE and SP Sephadex ion exchangers.
TYPES OF CHROMATOGRAPHY
1. Separation on the basis of size- Gel
Filtration chromatography:Proteins of different sizes are separated on a column in which the stationary phase is an polymerized agarose or acrylamide bead, which contain pores of various sizes. A smaller protein in the mobile phase (aqueous buffered solution) may enter the pores in the bead, while a larger protein may not, due to size restriction. The result is that a larger fraction of the overall volume of the column is available to the smaller protein, which thus spends a longer time on the column and is eluted by the mobile solvent after the larger protein. The shape of the protein (not only its size) also determines whether the protein will enter the pores. We used this technique to separate dye encapsulated in liposomes from free dye in Lab 1.
Lets consider in more depth the theory of gel filtration chromatography. Several different column volumes can be defined as shown below.

A solute elutes from the column in a broad peak. If the sample volume applied to the column is very small compared to Vt, the volume at which a solute elutes, Ve, is considered to be the center of the elution peak. This is true when Vsample << Ve.
If we view this chromatography has a partitioning of solute between the mobile and stationary phases, we might be interested in what fraction of the stationary phase, Vi, a solute might partition into. Such a ratio would be given by:
K = (Ve-Vo)/Vt-Vo)
where Vt-Vo (=Vinside) represents 100% of the
stationary phase, where K is a distribution coefficient. Consider two
cases- A very large solute compared to the pore size: In this case Ve-Vo = 0 since Ve would be equal to Vo. (The solute wouldn't see any of the Vi.) In this case, K = 0. The solute would elute in the void volume of the column since it can't partition into any of the volume within the beads. All solutes of molecular weight greater than or equal to the smallest solute that can't enter the gel beads will all elute in the void volume. Hence solutes greater than this minimal size will co-elute from the column and not be separated. Vo is usually about 30-40% of the Vt.
- A very small solute compared to the pore size. In this case Ve-Vo = Vt - Vo, since Ve would be equal to Vt. (The solute would see all of the solvent) In this case, K = 1. Similar to above, all solutes of MW equal to or less than the largest solute that can partition into the entire volume within a bead will co-elute at a volume near Vt.

Size and shape relationships:
K depends on the size and shape of the
solute. The size and shape of an object determines is flow properties in a
fluid. Frictional resistance (a force which has the opposite direction as
the velocity, another vector quantity, can be shown to be proportional to the
velocity.
Ff a
v or Ff = - fv
where f is the frictional coefficient, which
depends on shape. Clearly, the bigger the object, the more frictional
resistance to movement. For a sphere it can be shown that:
f = 6phRs
where h
is the viscosity (measure of the resistance to flow of a liquid - water has a
low viscosity, real maple syrup a high viscosity), and Rs (Stokes
radius) is the radius of the hydrated sphere (the larger Rs, the
larger the frictional coefficient, the larger the Ff which resists
motion). For an irregularly shaped object ,
the Stokes radius is the radius of a sphere that would have the same frictional
coefficient as the object. Hence the Rs for a protein molecule
that was not spherical in shape would be much larger than the Rs for
another protein molecule of identical molecular weight that was
spherical. Hence the Ve and the K value for a
solute on a gel filtration column would best be related to the Stokes radius,
since Rs values takes into account both size and shape.
It can be shown that:
Rs a
erf-1 (1-K) or Rs
= A + B erf-1 (1-K)
What would a plot of Rs vs erf-1
(1-K) look like?
For globular proteins, you
can also show that
-
K = -A log (MW) + B or more rigorously
-
K = -A' log Rs + B'
-
log Rs a log MW of the protein, and that, more practically,
-
a plot of log MW vs either K or Ve is linear with a negative slope.
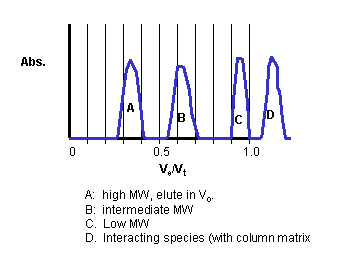
Hence gel filtration can be used to determine the MW of an unknown
spherical (globular) proteins when compared to a standard curve generated used
other globular proteins of known MW. In Lab 1 we separated liposomes-encapsulated
dye from free dye. That separation was trivial since it was equivalent in
difficult to separating elephants from mosquitoes. It is much more
difficult to separate proteins of similar size, a problem often faced in the
lab.
The chromatography resin in this type of chromatography consist of an agarose, acrylamide, or cellulose resin or bead which is derivatized to contain covalently linked positively or negatively charged groups. Proteins in the mobile phase will bind through electrostatic interactions to the charged group on the column. In a mixture of proteins, positively charged proteins will bind to a resin containing negatively charged groups, like the carboxymethyl group, CM (-OCH2COO-) or sulfopropyl, SP, (-OCH2CH2CH2SO3-) while the negatively charged proteins will pass through the column. The positively charged proteins can be eluted from the column with a mobile phase containing either a gradient of increasing salt concentration or a single higher salt concentration (isocratic elution). The most positively charged protein will be eluted last, at the highest salt concentration. Likewise, negatively charged proteins will bind to a resin containing positively charged groups, like the diethylaminoethyl group, DEAE (-OCH2CH2NH(C2H5)2+) or a quarternary ethyl amino group, QAE, and can be separated in an analogous fashion. In chromatofocusing, the mobile phase contains a gradient in pH, and bound proteins are eluted when the pH reaches the isoelectric point of the protein, at which the proteins have no net charge. In this lab, you will use QAE and SP Sephadex ion exchangers.
No comments:
Post a Comment