|
SOLUBILITY PRODUCT and THE COMMON ION EFFECT
This page looks at the common ion effect related to solubility
products, including a simple calculation. You need to know about
solubility products and calculations involving them before you read this
page. What is the common ion effect? I need to look again at a simple solubility product calculation, before we go on to the common ion effect. The solubility of lead(II) chloride in water Lead(II) chloride is sparingly soluble in water, and this equilibrium is set up between the solid and its ions in solution: If you just shook up some solid lead(II) chloride with water, then the solution would obviously contain twice as many chloride ions as lead(II) ions. The expression for the solubility product and its value are given by: | |
|
Note: I have no confidence in the accuracy of this value. There are serious discrepancies between the values from different sources. But that makes no difference to the discussion. | |
|
For comparison purposes later, I need to work out the lead(II) ion concentration in this saturated solution. If the concentration of dissolved lead(II) chloride is s mol dm-3, then: Put these values into the solubility product expression, and do the sum.  So the concentration of lead(II) ions in the solution is 1.62 x 10-2 mol dm-3 (or 0.0162 mol dm-3 if you prefer). What happens if you add some sodium chloride to this saturated solution? Now we are ready to think about the common ion effect. Sodium chloride shares an ion with lead(II) chloride. The chloride ion is common to both of them. This is the origin of the term "common ion effect". Look at the original equilibrium expression again: What would happen to that equilibrium if you added extra chloride ions? According to Le Chatelier, the position of equilibrium would shift in order to counter what you have just done. In this case, it would tend to remove the chloride ions by making extra solid lead(II) chloride. | |
|
Note: Actually, of course, the concentration of lead(II) ions in the solution is so small to start with, that only a tiny proportion of the extra chloride ions can be converted into solid lead(II) chloride. | |
|
The lead(II) chloride will become even less soluble - and, of course,
the concentration of lead(II) ions in the solution will decrease. Something similar happens whenever you have a sparingly soluble substance. It will be less soluble in a solution which contains any ion which it has in common. This is the common ion effect. A simple calculation to show this Suppose you tried to dissolve some lead(II) chloride in some 0.100 mol dm-3 sodium chloride solution instead of in water. What would the concentration of the lead(II) ions be this time? As before, let's call the concentration of the lead(II) ions s. Now the sum gets different. This time the concentration of the chloride ions is governed by the concentration of the sodium chloride solution. The number of ions coming from the lead(II) chloride is going to be tiny compared with the 0.100 mol dm-3 coming from the sodium chloride solution. In calculations like this, you can always assume that the concentration of the common ion is entirely due to the other solution. This makes the maths a lot easier. In fact if you don't make this assumption, the maths of this can become impossible to do at this level. So we assume: The rest of the sum looks like this: 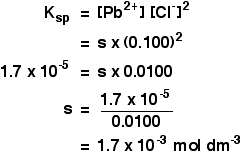 Finally, compare that value with the simple saturated solution we started with: Original solution: Solution in 0.100 mol dm-3 NaCl solution: The concentration of the lead(II) ions has fallen by a factor of about 10. If you tried the same sum with more concentrated solutions of sodium chloride, the solubility would fall still further. Try it yourself with chloride ion concentrations of 0.5 and 1.0 mol dm-3. | |
Tuesday, 24 September 2013
SOLUBILITY PRODUCT and THE COMMON ION EFFECT
Subscribe to:
Post Comments (Atom)
No comments:
Post a Comment